
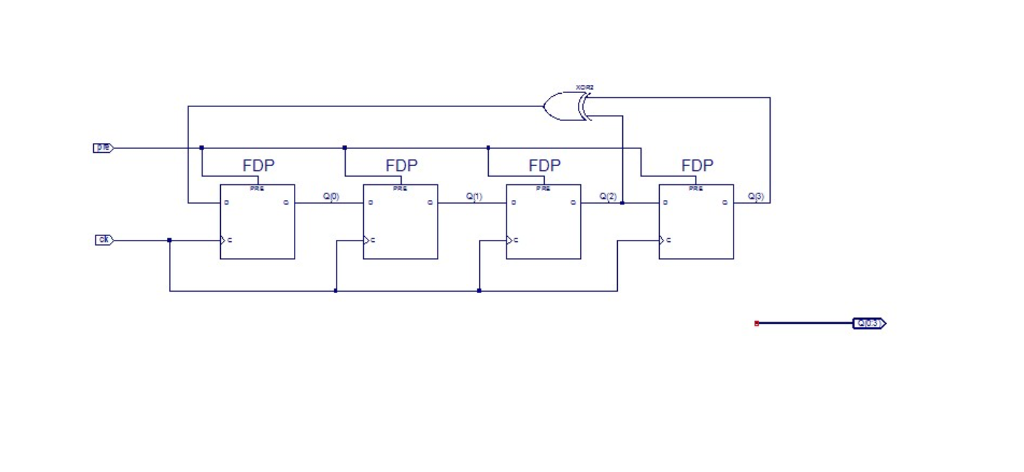

In that case the Linear Predictor tries to determine the next number in the sequence using a linear combination of previous samples. LFSRs are closely related to Linear Predictors often used in DSP. The A5/2 cipher has been broken and both A5/1 and E0 have serious weaknesses. Important LFSR-based stream ciphers include A5/1 and A5/2, used in GSM cell phones, E0, used in Bluetooth. Once the LFSR is known, who whole output stream is known. Given a small piece of the LFSR output stream, an identical LFSR of minimal size can be easily recovered using the Berlekamp-Massey algorithm. Unfortunately, an LFSR is a linear system, which makes cryptanalysis easy. LFSRs have been used in the past as pseudo-random number generators for use in stream ciphers due to their simplicity.
This page will try to explain Linear Feedback Shift Registers (LFSRs) and how to generate a minimal length LFSR given a bitstream.


 0 kommentar(er)
0 kommentar(er)
